Video Demonstrations

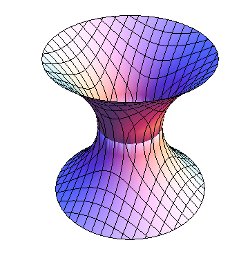This wormhole consists of curved empty space. The wormhole throat collapses in on itself so fast that nothing, not even a beam of light, has enough time to get through. Anything attempting to pass through such a wormhole would get caught in the “pinch-off” and would be crushed by infinite gravitational forces. Like black holes, nontraversable wormholes also have event horizons. These features make them unsuitable for interstellar travel. See the discussion at the beginning of chapter 9.

Any curved surface can be considered flat over a small enough region. The demonstration shows a portion of a sphere with a blue region which is locally flat, i.e., small in size compared to the radius of the sphere. As we reduce the size of the sphere, it becomes “more curved,” i.e., has a smaller radius of curvature, than a larger sphere. The demonstration shows that the region over which a surface can be considered flat is smaller the more curved the surface. See the discussion at the beginning of chapter 12.

The shaded region represents the worldtube of an Alcubierre warp bubble. The light cones inside the bubble are distorted relative to the light cones outside the bubble. The line at the center of the shaded region represents the worldline of a spaceship at the center of the bubble. Although the ship can travel at faster-than-light speed with respect to observers outside the bubble, the worldline of the spaceship always lies within its local light cone. Therefore the ship always travels slower than the local speed of light, i.e., the speed of light inside the bubble. The demo shows what the light cones look like for various (constant) bubble velocities. Refer to the discussion of figure 9.2. (Demo by Tim Ouellette, based on a program by Jim Hartle.)

Here we have end and side views of a flat two-dimensional (one time and one space dimension) spacetime which has been rolled up into a cylinder. The time axis is a green circle that runs around the cylinder’s axis. Despite being rolled into a cylinder, the spacetime is still flat and so the light cones have their original orientation. (Note that if you roll a piece of paper into a cylinder, the sum of the angles of a triangle drawn on it is still 180 degrees, unlike on the curved surface of a sphere.) The heavy black line represents a surface (or in this case, a line) of simultaneity. The green curve represents the worldline of an observer in this universe. At the beginning of the demonstration, we show an observer at rest who simply goes around the cylinder in time, experiencing all the same moments again and again. As the video proceeds, we show the observer moving; notice that his worldline spirals around the cylinder. His worldline will then intersect the heavy black line multiple times. This means that at any one time there will be multiple copies of the observer present at different locations in space, each of a different age according to the observer’s own proper time. For more strange features of this spacetime, see the discussion at the beginning of chapter 13.

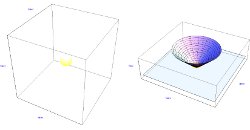The frame on the left shows an expanding sphere of light in the three dimensions of space. The frame on the right shows the same thing in spacetime, with one spatial dimension suppressed (i.e., the expanding yellow circle is really a sphere). Think of the analogy of dropping a pebble in a pond and watching a ripple spreading out from the center. For more details, see the discussion of the light cone in chapter 4.

Chris Van Den Broeck suggested a way to drastically reduce the total amount of negative energy required for an Alcubierre warp bubble. He proposed putting a spaceship at the center of an inflated region of space, which is attached to the bubble wall by a very narrow neck. The size of the bubble might only be about the size of a proton, while the region of space contained inside could be enormous. As the demonstration proceeds, notice that the inflated region of space at the bottom (the “bottle”) can become very large while the warp bubble at the top of the neck remains small. See the discussion in chapter 12.

The graphic shows the energy density for a squeezed vacuum state of a massless field as a function of time. As the demonstration proceeds, the level of squeezing is increased. Note that the red pockets of negative energy get larger in magnitude (i.e., deeper troughs) but their duration in time decreases (i.e., the red regions get thinner). At the same time, the blue regions of positive energy greatly increase. These behaviors are in accord with the quantum inequalities. See the discussion in chapter 11.

The graphic shows the energy density for a squeezed vacuum state of a massive field as a function of time. As the demonstration proceeds, the level of squeezing is increased. The red pockets of negative energy get larger in magnitude (i.e., deeper troughs) but their duration in time decreases (i.e., the red regions get thinner). At the same time, the blue regions of positive energy greatly increase. However, unlike the case of the massless field, continued squeezing eventually causes the negative energy regions to disappear, leaving only the positive energy. It is harder to produce negative energy with massive fields than with massless fields because, in the massive case, one must also overcome the positive rest mass of the field particles. See the discussion in chapter 11.

The long horizontal elevator car, depicted on the left, falls freely in the earth’s gravitational field. At each end of the car is a ball bearing. The balls are falling toward the center of gravitational attraction, the center of the earth. Since their paths are not parallel, there is a part of the earth’s gravitational force which is directed along the line joining their centers. As a result, the ball bearings feel a force pushing them together. Now consider the freely falling vertically-oriented car on the right, which also contains two ball bearings. The lower ball is slightly closer to the center of the earth than the upper ball and so will feel a slightly larger gravitational force. Therefore, it will fall slightly faster than the upper ball. As time goes on the upper ball will get left behind, and the two balls experience a stretching force which pulls them apart. See the discussion accompanying figures 8.5 and 8.6.

A light clock at rest in frame S′ moves with constant velocity to the right, relative to several synchronized clocks at rest in frame S. According to the principles of relativity, a light beam (here illustrated by a photon—a particle of light) travels at the same speed in both frames. As seen in S, the photon associated with the moving light clock travels a longer distance at the same speed to complete one round trip. Therefore, clocks S in register more time than the moving clock, so observers in will say that the moving clock in S′ is running slow compared to their clocks. Refer to Appendix 5 for more details.

Refer to figure 8.9 and its associated discussion. This demonstration shows the formation of a so-called trapped surface. The shrinking circle represents a star collapsing to form a black hole. As the star shrinks, the light cones associated with its surface tilt over, relative to the light cones of events that are far from the star. If the outgoing legs of the surface light cones become vertical, as in figure 8.9, an event horizon forms around the collapsing star. When they tilt even farther, toward the center of the star, no light from the star can escape to outside observers, and spacelike surfaces inside the horizon become “trapped.” A black hole has formed. Anything that falls across the horizon must inexorably fall toward the center. You can think of this graphic as a superposition of horizontal slices of figure 8.9.

Consider two twins, one who remains on earth and one who travels in a spaceship to a distant star and back. The vertical purple line represents the worldline of the stay-at-home twin, and the earth time elapsed for this twin is displayed in purple near the top of the demonstration. The bent green line represents the worldline of the travelling twin on the spaceship, and the elapsed time as measured on the ship is displayed in green. (Here, for simplicity, we have ignored periods of acceleration and deceleration. That is, we have assumed that the travelling twin travels at constant velocity out to the star, immediately turns around, and travels at constant velocity back to earth. This is why the green line appears sharply bent instead of curved.) The horizontal axis measures the distance from the earth in light years. The speed of the travelling twin is given in red at the top of the diagram, expressed as a fraction of the speed of light. By moving the playback slider on the demonstration, you can vary the velocity of the travelling twin. As the slope of the travelling twin’s worldline gets closer to 45 degrees, the speed of the ship gets closer to the speed of light. Notice that therefore less and less time passes on the ship, compared to the time elapsed on earth, as can be seen at the top of the diagram. See the discussion in chapter 5.